Centripetal Force Calculator
Use our centripetal force calculator to solve force, mass, radius, or velocity.
Centripetal Force:
Centripetal Force Formula
Mass Formula
Radius Formula
| Tangential Velocity: | |
| Angular Velocity: |
Tangential Velocity Formula
Angular Velocity Formula
On this page:
Joe is the creator of Inch Calculator and has over 20 years of experience in engineering and construction. He holds several degrees and certifications.
How to Calculate Centripetal Force
Whether it’s a car turning around a curve, a satellite orbiting the Earth, or a stone tied to a string being swung in a circle, centripetal force is a fundamental concept in how objects move in a circular motion. Centripetal force refers to the force that is necessary to keep an object moving in a circular path and is directed towards the center of the circle.
Without this force, an object would continue moving in a straight line, according to Newton’s First Law of Motion. The term “centripetal” comes from Latin words meaning “center seeking”, which aptly describes the direction of the force.
You can calculate centripetal force using a formula.
The Centripetal Force Formula
The formula for calculating centripetal force F is:
F = mv² / r
Where:
F = centripetal force (in N)
m = mass of the object moving in the circle (in kg)
v = velocity of the object (in m/s)
r = radius of the circular path (in m)
The centripetal force formula states that the force F is equal to the mass of the object m times its tangential velocity v squared, divided by the radius of its path r.
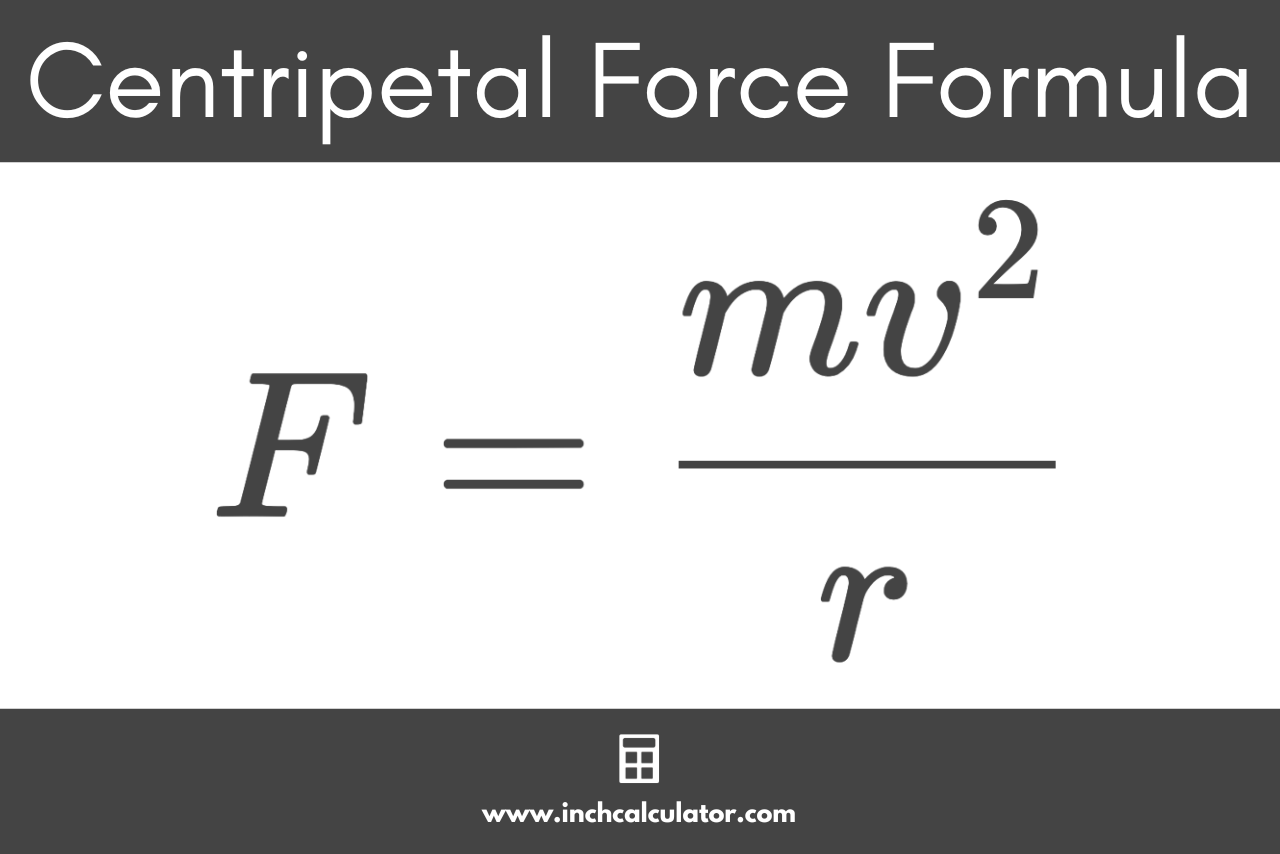
This equation shows that the centripetal force required to keep an object moving in a circular path increases with the square of the velocity and inversely with the radius of the circle. Therefore, an increase in speed or a decrease in the radius requires a larger centripetal force to maintain circular motion.
For example, let’s consider a car with a mass of 1,000 kg moving at a speed of 20 m/s around a circular track with a radius of 50 meters. Let’s find the centripetal force acting on the car.
F = 1,000 kg × 20 m⁄s² / 50 m
F = 8,000 N
Thus, the centripetal force required to keep the car moving in this circular path is 8,000 Newtons directed toward the center of the circle.
Centripetal Force vs. Centrifugal Force
It’s important to distinguish between centripetal and centrifugal forces, since they are often confused. Centripetal force, as discussed above, is the real force that acts on an object moving in a circle, directed towards the center of the circle. It is the necessary force that keeps the object in circular motion.
Centrifugal force, on the other hand, is not a real force; rather, it is a perceived force that appears to act outward on an object when viewed from a rotating reference frame. This is a fictitious force that arises due to the inertia of the object moving in a circular path; it is the object’s tendency to move straight, as experienced by an observer moving with the object.
For example, when you are turning in a car, you might feel like you are being pushed outwards against the door. This sensation is due to the centrifugal force.
Said another way, centripetal force is the actual force that keeps an object in a circular path, directed towards the center, while centrifugal force is the apparent force that seems to push the object outwards, felt by observers within the rotating frame.