Degrees to Radians Converter
Enter the angle in degrees below to convert it to radians.
Result in Radians:
360° = 2π
Do you want to convert radians to degrees?
On this page:
Joe is the creator of Inch Calculator and has over 20 years of experience in engineering and construction. He holds several degrees and certifications.
Ethan has a PhD in astrophysics and is currently a satellite imaging scientist. He specializes in math, science, and astrophysics.
How to Convert Degrees to Radians
To convert a measurement in degrees to a measurement in radians, you need to use a conversion formula. Since pi radians are equal to 180°, you can use the following formula to convert degrees to radians:
radians = degrees × π / 180
In other words, the angle in radians is equal to the angle in degrees times pi, divided by 180.
To use this formula, start by substituting the angle in degrees into the formula. Then, move the degrees to the top of the fraction, and finally, simplify the fraction and evaluate.
radians = 5° × π / 180
radians = 1 × π / 36
radians = π / 36
Alternate Degree to Radian Formula
If you want to convert degrees to radians without using pi, multiply the angle by the following conversion ratio: 0.017453 radians/degree.
Since one degree is equal to 0.017453 radians, you can use this simple formula to convert:
radians = degrees × 0.017453
The angle in radians is equal to the angle in degrees multiplied by 0.017453.
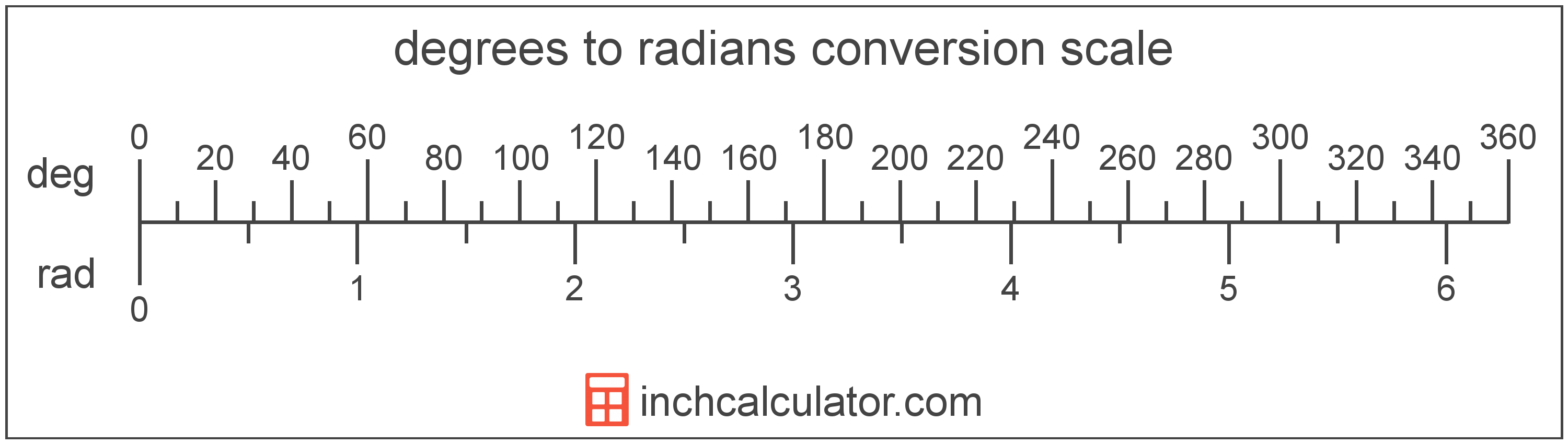
Degrees and radians are both units used to measure angle. Keep reading to learn more about each unit of measure.
What Is a Degree?
A degree is a measure of angle equal to 1/360th of a revolution, or circle.[1] The number 360 has 24 divisors, making it a fairly easy number to work with. There are also 360 days in the Persian calendar year, and many theorize that early astronomers used 1 degree per day.
The degree is an SI accepted unit for angle for use with the metric system. A degree is sometimes also referred to as a degree of arc, arc degree, or arcdegree. Degrees can be abbreviated as °, and are also sometimes abbreviated as deg. For example, 1 degree can be written as 1° or 1 deg.
Degrees can also be expressed using arcminutes and arcseconds as an alternative to using the decimal form. Arcminutes and arcseconds are expressed using the prime (′) and double-prime (″) characters, respectively, although a single-quote and double-quote are often used for convenience.
One arcminute is equal to 1/60th of a degree, and one arcsecond is equal to 1/60th of an arcminute.
Protractors are commonly used to measure angles in degrees. They are semi-circle or full-circle devices with degree markings allowing a user to measure an angle in degrees. Learn more about how to use a protractor or download a printable protractor.
Learn more about degrees.
What Is a Radian?
A radian is the measurement of angle equal to the length of an arc divided by the radius of the circle or arc.[2] 1 radian is equal to 180/π degrees, or about 57.29578°. There are about 6.28318 radians in a circle.
The radian is the SI derived unit for angle in the metric system. Radians can be abbreviated as rad, and are also sometimes abbreviated as c, r, or R. For example, 1 radian can be written as 1 rad, 1 c, 1 r, or 1 R.
Radians are often expressed using their definition. The formula to find an angle in radians is θ = s/r, where the angle in radians θ is equal to the arc length s divided by the radius r. Thus, radians may also be expressed as the formula of arc length over the radius.
Radians are also considered to be a "unitless" unit. That is, when multiplying or dividing by radians, the result does not include radians as part of the final units.
For example, when determining the length of an arc for a given angle, we use the formula above, rearranged to be s = θr. If θ is in radians and r is in meters, then the units of s will be meters, not radian-meters. If θ were in degrees, however, then s would have units of degree-meters.
Learn more about radians.
Degree to Radian Conversion Table
| Degrees | Radians (expression) | Radians (decimal) |
|---|---|---|
| 0° | 0 rad | 0 rad |
| 15° | π/12 rad | 0.261799 rad |
| 30° | π/6 rad | 0.523599 rad |
| 45° | π/4 rad | 0.785398 rad |
| 60° | π/3 rad | 1.047198 rad |
| 90° | π/2 rad | 1.570796 rad |
| 120° | 2π/3 rad | 2.094395 rad |
| 150° | 5π/6 rad | 2.617994 rad |
| 180° | π rad | 3.141593 rad |
| 270° | 3π/2 rad | 4.712389 rad |
| 360° | 2π rad | 6.283185 rad |
References
- Collins Dictionary, Definition of 'degree', https://www.collinsdictionary.com/us/dictionary/english/degree
- International Bureau of Weights and Measures, The International System of Units, 9th Edition, 2019, https://www.bipm.org/documents/20126/41483022/SI-Brochure-9-EN.pdf