Depreciation Calculator
Calculate the depreciation of an asset using the straight line, declining balance, double declining balance, or sum-of-the-years’ digits method.
Depreciation Schedule:
| Year | Depreciation Amount |
|---|---|
| 2024 | $19,000 |
| 2025 | $19,000 |
| 2026 | $19,000 |
| 2027 | $19,000 |
| 2028 | $19,000 |
On this page:
- Calculator
- How to Calculate Depreciation
- What is Depreciation?
- How to Calculate Straight-Line Depreciation
- Straight-Line Depreciation Formula
- How to Calculate Declining Balance
- Declining Balance Formula
- Double-Declining Balance Formula
- How to Calculate Sum-of-the-Years’ Digits
- Sum-of-the-Years’ Digits Formula
- Which Depreciation Method Should I Use?
- Frequently Asked Questions
- References
Andrew holds a Bachelor's degree in Finance and a Bachelor's degree in Political Science from the University of Colorado and specializes in finance, real estate, and life insurance.
Monica Greer holds a PhD in economics, a Master's in economics, and a Bachelor's in finance. She is currently a senior quantitative analyst and has published two books on cost modeling.
Laura started her career in Finance a decade ago and provides strategic financial management consulting. She has an MBA in Finance and a Bachelor's in Economics.
How to Calculate Depreciation
Most physical goods—machinery, rental properties, equipment, and even office supplies—have a limited useful life. And if these goods and materials are essential for conducting business, they will eventually need to be replaced.
Suppose there is a piece of equipment that costs $100 and the useful estimated life of this piece of equipment is 10 years.
While it is quite possible that the business paid for this piece of equipment all at once (i.e., they did not need to finance it), that business might still consider spreading these costs over time. Typically, items under $2,500 are not depreciated and are instead taken as an expense all at once.
Depreciation is a broad finance and accounting term used to describe various ways of distributing these costs. As we will further illustrate throughout this article, there are several different depreciation strategies that can make sense in certain situations.
What is Depreciation?
It is important to remember that depreciation is a cost allocation strategy, and not what a business is paying for an asset each month. Depreciation is an accounting strategy that helps spread the total cost of acquiring an asset over that asset’s useful lifetime.
If an asset’s book value is 90% of its original value, that doesn’t necessarily mean you could sell the product for that price on the open market. Instead, this simply means that 10% of that asset’s value has been accounted for.
Depreciation helps make it easier for businesses and other organizations to control their expenses. This can be useful in a variety of situations, such as determining the entire value of a company or minimizing tax obligations.
How to Calculate Straight-Line Depreciation
One of the most common depreciation strategies is straight-line depreciation. This strategy will evenly spread an asset’s cost over its entire life. In other words, depreciation will be linear.
To calculate straight-line depreciation, you need to know the cost of the asset, its estimated service life, and its salvage value. The salvage value is the amount you could sell the asset or its parts for once its useful life has been exhausted.
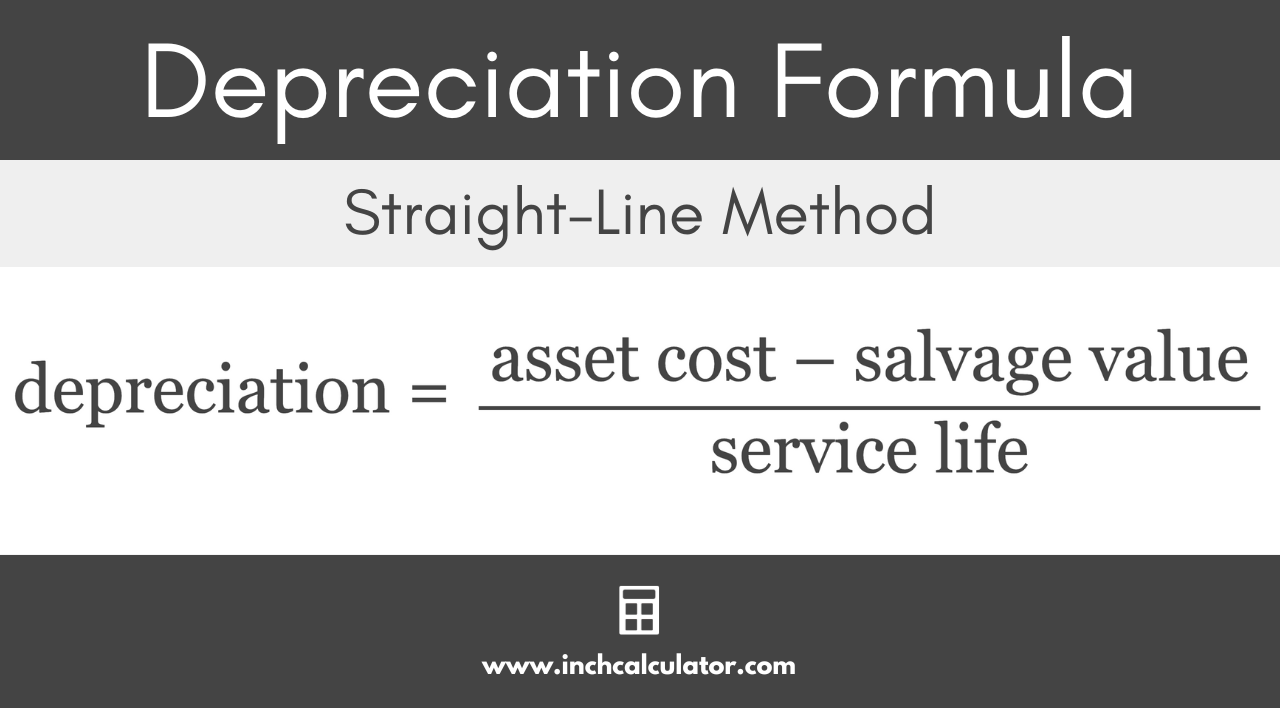
Straight-Line Depreciation Formula
The formula for straight-line depreciation is:[1]
depreciation = asset cost – salvage value / service life
Using the straight-line method, depreciation is equal to the asset cost minus the salvage value, divided by the service life.
Suppose an asset costs $100, its estimated salvage value is $10, and its estimated useful life is 10 years:
depreciation = $100 – $10 / 10
depreciation = $9
In this case, the business can account for a $9 depreciation expense annually for the asset. Of course, if the asset is still useful after ten years, the business can continue using it, but they cannot continue accounting for its depreciation.
How to Calculate Declining Balance
The declining balance depreciation method assumes that the asset will lose value at a faster rate towards the beginning of its useful life than it will at the end of its useful life (think about how a new car loses much of its value as soon as it drives off the lot).
In order to calculate the declining balance formula, you will need to incorporate a corresponding depreciation factor.
Declining Balance Formula
The formula for declining balance depreciation is:[2]
depreciation = value × factor × 1 / service life
Where:
value = depreciable value at the beginning of the year
service life = years of useful life
factor = depreciation factor (see below for tips to find this)
Using the declining balance method, depreciation is equal to the value times the depreciation factor times 1 divided by the service life.
In the formula above, the depreciation factor you choose will depend on how quickly you believe the asset will lose its value. The larger the factor, the more quickly the asset’s value will be depreciated in the beginning of its life.
This strategy is useful for assets that decline in value quickly, such as computers or other technology that rapidly becomes obsolete. In some cases, there might be an industry standard available that you can use as a baseline.
In other cases, you might simply want to depreciate at double the linear rate using the double-declining balance formula below.
Double-Declining Balance Formula
The double-declining balance formula is shown below:
depreciation = value × 2 × 1 / service life
Where:
value = depreciable value at the beginning of the year
service life = years of useful life
Using the double-declining balance method, depreciation is equal to the value times 2 times 1 divided by the service life.
Suppose that the value of the product at the beginning of the year is $50 and you believe the product has 4 more useful years remaining. In this case, all you’d need to do is:
Depreciation = $50 x 2 x (1/4)
Depreciation = $25
The double-declining balance formula calculates that the depreciation entry for that year would be $25.
How to Calculate Sum-of-the-Years’ Digits
The sum-of-the-years’ digits depreciation method is another common depreciation strategy that places a higher emphasis on the early years and less emphasis on the later years. As the years go on, the depreciation percentage will change accordingly.
Sum-of-the-Years’ Digits Formula
The sum-of-the-years’ digits formula is:
depreciation = (asset cost – salvage value) × factor
Where:
n = service life in years
factor = years of service life remaining divided by the sum of each year’s digits (see example below)
year 1 factor = n / 1 + 2 + … + n
year 2 factor = n – 1 / 1 + 2 + … + n
final year factor = 1 / 1 + 2 + … + n
Which Depreciation Method Should I Use?
The depreciation method that makes the most sense for you will depend on several different factors. To start with, you might want to consider the value of a product that may deteriorate over time.
For example, the drop in value between a new product and a product that is one year old will almost always be greater than, say, the drop in value between years 2 and 3.
You might also want to think about the likelihood that you will sell the product before its useful life has been exhausted.
In most cases, the straight-line method will be easiest to apply and will make the most sense. Regardless of which method you use, be sure to use depreciation in order to distribute the cost of assets over time.
For example, let’s compare these depreciation methods using the following parameters:
- Asset Cost = $100,000
- Salvage Value = $5,000
- Service Life = 5 years
- Depreciation Factor (DB method only) = 2.06
- Convention = Full-Month
- Placed in Service = January 2022
The resulting depreciation schedules look like this:
| Year | Depreciation Amount for Method | |||
|---|---|---|---|---|
| Straight-Line | Declining Balance (factor = 2.06) | Double-Declining Balance | Sum-of-the-Years’ Digits | |
| 2022 | $19,000 | $41,200 | $40,000 | $31,667 |
| 2023 | $19,000 | $24,226 | $24,000 | $25,333 |
| 2024 | $19,000 | $14,244 | $14,400 | $19,000 |
| 2025 | $19,000 | $8,376 | $8,640 | $12,667 |
| 2026 | $19,000 | $4,925 | $5,184 | $6,333 |
| Total | $95,000 | $92,971 | $92,224 | $95,000 |
So, two methods attain the fully depreciated status:
$100,000 – $95,000 = $5,000 (salvage value)
But what about the other two methods, which haven’t depreciated enough to attain this status?
In cases where the declining balance method will not fully depreciate an asset over its service life there are a few options.
The most commonly adopted option is to switch the depreciation method to use the straight-line method later in the asset’s life.
Another option is to use a higher depreciation factor to further accelerate the depreciation ensuring the asset is fully depreciated.
If the asset is not fully depreciated then it will have a higher book value than its salvage value, ultimately resulting in a lower tax benefit.
You might also be interested in our appreciation calculator to figure out the increase in value of an asset over time.
Frequently Asked Questions
What does depreciation do?
Depreciation helps spread the total cost of acquiring an asset over that asset’s useful lifetime, thus allowing companies to spread out their costs instead of recognizing them all at once, which could skew their true profitability.
Why is depreciation important?
Depreciation is important because it allows companies to spread the cost of an asset over that asset’s useful life. This allows companies to reduce their tax bill by recognizing the lost value of an asset over time, as well as show investors and stakeholders an accurate depiction of company performance.
Without depreciation, company performance may be skewed with an asset’s full expense being recognized in one period, instead of the over the time period it’s actually being used.
Why is depreciation an expense?
Depreciation is an expense because it is a normal business operating expense: as an asset is used over time and has more wear and tear, the value of that asset drops. Depreciation is recognized on the income statement, which is where normal business operating expenses are shown.
References
- Chamber of Commerce, The Small Business's Guide to Straight Line Depreciation, https://www.chamberofcommerce.org/small-businesss-guide-to-straight-line-depreciation
- Internal Revenue Service, Publication 946, How To Depreciate Property, https://www.irs.gov/publications/p946