Discounted Cash Flow Calculator
Calculate the discounted present value (DPV) of an investment using the discounted cash flow (DCF) model.
Discounted Present Value (DPV):
| Growth Value: | $ |
| Terminal Value: | $ |
| Total Value: | $ |
On this page:
Joseph Rich holds a Master's degree in finance and a Bachelor's degree in economics. He specializes in economics and investing analysis.
Monica Greer holds a PhD in economics, a Master's in economics, and a Bachelor's in finance. She is currently a senior quantitative analyst and has published two books on cost modeling.
How to Calculate a Discounted Cash Flow Analysis
Discounted cash flow (DCF) analysis is a method that can be used to value a particular investment based on its expected future cash flows. This can be used for valuing a stock or a particular project a company is considering.
In the case of a stock, future cash flows would be dividends. If a company is considering whether or not to invest in a project, the cash flows can be cash coming into the business, such as with a machine that improves the product and increases revenue.
Cash flows can also be in the form of savings, such as with video conferencing technology that may reduce the expenses related to office space.
A DCF analysis takes all future cash flows and discounts them using the discount rate or weighted average cost of capital (WACC). An investor/company can choose whether a discount rate or WACC is most appropriate for their analysis.
Discount Rate and WACC
The discount rate and weighted average cost of capital are the rates that investors and businesses use to discount future cash flows.
The discount rate is the rate that is used for investments of similar risk. If an investor typically earns 8% on his investments, that is the discount rate he will use in evaluating potential new investments.
The level of risk is different for different types of investments, so using a discount rate that does not align with the particular investment could provide misleading results.
The WACC is the weighted average of the cost of debt and the cost of equity. This is the return that business owners would require their business to make with an investment. If the investment doesn’t return at least as much as its WACC, the investment should be rejected.
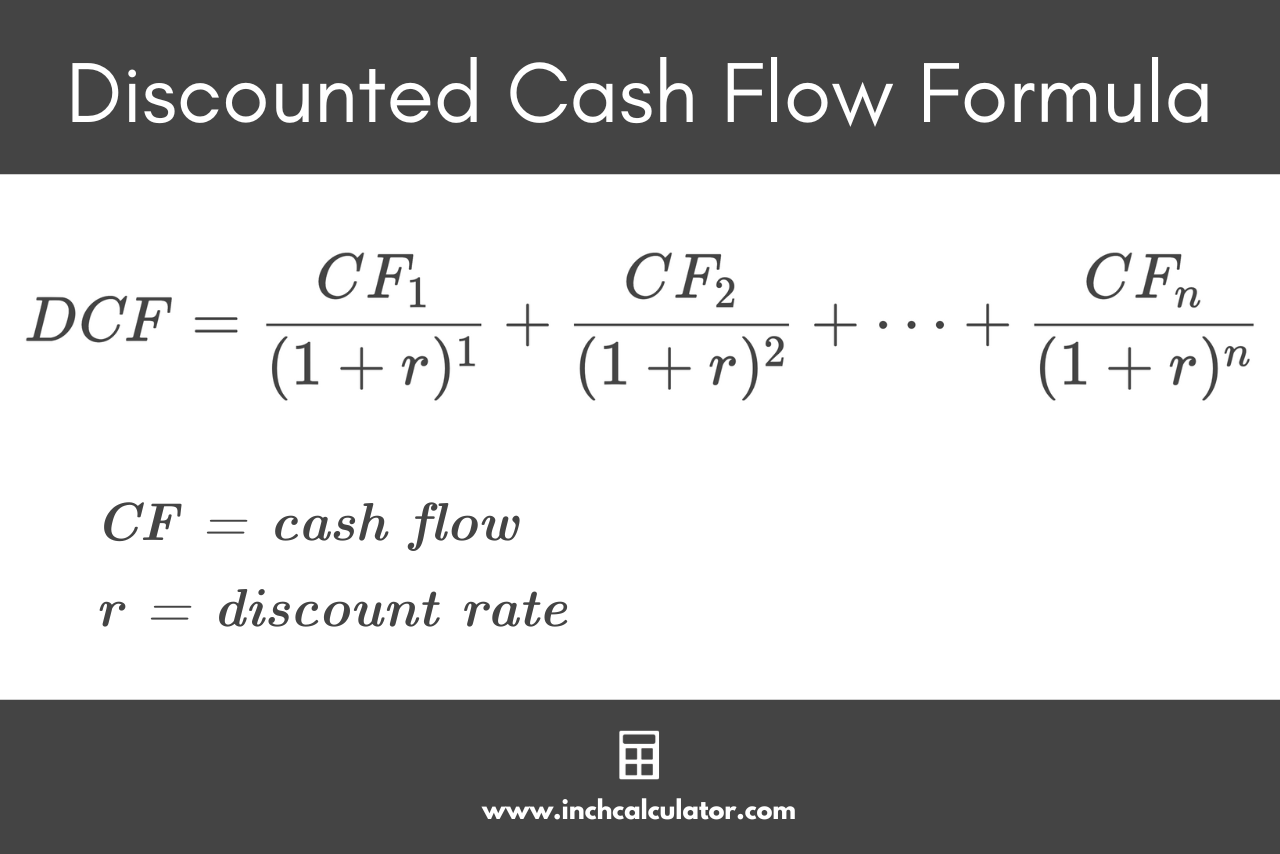
Discounted Cash Flow Formula
The discounted cash flow formula below shows how the discount rate and WACC are applied.
The discounted cash flow formula is shown below:[1]
where:
DCF = discounted cash flow
CF = cash flow for a given period
r = discount rate
As the formula shows, each future cash flow is discounted by 1 plus the discount rate or WACC raised to the power of the time period. After each future cash flow is discounted, all the cash flows are summed up to arrive at the discounted cash flow.
Discounted Cash Flow Analysis Example
For example, to help explain how the discounted cash flow calculation works, let’s say an investor is deciding whether or not to buy a stock. The company will pay a $1.00 dividend next year. Based on historical data, he anticipates the dividend will then grow by 2% for 20 years and then by 1% for 20 years after that.
The investor will then plan on selling the stock. They typically make 8% on their investments, so this will be the discount rate.
Since this involves a period of 40 years, it is much simpler to plug these numbers into the calculator than work the formula by hand. You will need the cash flow and discount rate plus the following variables for the calculation:
- Growth Rate: The rate at which the cash flow is initially projected to grow each year.
- Growth Period: The number of years for this initial growth period.
- Terminal Rate: The rate at which the cash flow is projected to grow each year after the initial growth period.
- Terminal Period: The number of years the cash flow will grow at the terminal rate.
In this example, the cash flow will be $1. The discount rate is 8%. The growth rate is 2% and the growth period is 20 years. The terminal rate is 1% and the terminal period is 20 years.
After plugging these numbers into the discounted cash flow calculator above, we get a total DCF of $14.98. If the stock is trading for anything less than $14.98, the investor would want to purchase it because the present value of all future cash flows is greater than the price of the stock.
But, if it is trading for more than $14.98, the investor should not buy the stock.
Now let’s look at an example of a company deciding whether or not it wants to move forward with a project. It anticipates making a $100,000 investment in a technology that will increase its revenues immediately by $25,000.
For the next 2 years after that, the increase in revenue will be 5% higher than the prior year. Then for the next 3 years, revenue will only be 2% higher than the previous year. The company has a WACC of 10%. Is this a smart investment?
Since this is only for 4 years, we can use either the discounted cash flow formula or the calculator above. Let’s use the formula first, and then you can confirm in the calculator. The table below gives the results.
| Period | Cash Flow | Discounted Cash Flow |
|---|---|---|
| 1 | 26,250.00 | 23,863.64 |
| 2 | 27,562.50 | 22,778.93 |
| 3 | 28,113.75 | 21,122.28 |
| 4 | 28,676.03 | 19,586.11 |
| 5 | 29,249.55 | 18,161.67 |
| Total | 139,851.82 | 105,512.62 |
Here again, we see that the investment is worth it. The DCF of $105.512.62 is greater than the initial investment of $100,000. If the DCF had been less than the initial investment, the company would not have gone through with the technology investment.
To find the breakeven point, use our IRR calculator. It shows what the discount rate would need to be to make the cash flows equal to the initial investment.
Discounted Cash Flow Limitations
While the DCF analysis is a helpful tool for planning purposes, there are a handful of limitations.
First, estimating forecasts, such as future cash flows and growth rates, far in advance is very difficult. So while it does provide an investor or business a framework to make a decision, whether or not the correct decision was made won’t be known until the end of the time period.
Also, not all companies pay a dividend. Newer companies typically won’t pay a dividend and instead will reinvest income back into the business. In this case, the DCF model computes a value of $0 for these companies. We know that newer companies aren’t worth $0, so a DCF analysis can’t be used for them.
Negative amounts can’t be used in the DCF analysis either. If a business expects to have to repair a machine after a certain number of years, this can’t be factored into the analysis. This limitation can also apply to an investment in which a business may reduce its dividend in a recession.
Discounted Cash Flow vs. Net Present Value
The discounted cash flow is the sum of all the discounted cash flows. The net present value (NPV) is what is left over after the initial investment is made. So the NPV = DCF – initial investment.
DCF by itself doesn’t give any direction on the decision to be made. The NPV does. If the NPV of a project or investment is greater than $0, the investment should generate a return.[2]
Under these assumptions, a return of at least the discount rate or WACC will be achieved. There are other calculators to calculate the rate of return, such as our ROI calculator and rate of return calculator.
If the NPV is less than $0, the investment should not be made. In an earlier example, we had a business trying to decide if it should invest $100,000 in new technology. The DCF was $105,512.62. Therefore, the NPV was positive $5,512.62 and the business should have made the investment.
Our NPV calculator is similar to the discounted cash flow calculator, but each of the cash flows needs to be entered. If there is only one future cash flow, then you can use a present value calculator, which will calculate what the value of that future cash flow is today, discounted at a certain rate for a period of time.
The larger the NPV, the better. This provides an additional margin for error in the forecasts.
References
- Paiano, F., Introduction to Investments - 4.3: The Discounted Cash Flow Model, LibreTexts Business, July 27, 2022, https://biz.libretexts.org/Bookshelves/Finance/Introduction_to_Investments_(Paiano)/02%3A_Chapter_2/04%3A_Fundamental_Analysis-_Valuation_Models/4.03%3A_New_Page
- Vo, E., Is Discounted Cash Flow the Same as Net Present Value?, The Hartford, September 14, 2022, https://sba.thehartford.com/finance/cash-flow/discounted-cash-flow-versus-net-present-value/