Wire Size Calculator

Calculate the minimum size of a wire or conductor needed for a circuit, or calculate the dimensions of the wire, including the diameter, cross-sectional area, and resistance given its gauge.

Conductor:

Wire Length:

Phase:

Supply Voltage:

Load Rating:

Allowable Voltage Drop:

Minimum Wire Size:

AWG

Expected Voltage Drop

Learn how we calculated this below

scroll down

Get Electrical Calculator on Google Play

Add this calculator to your site

How to Calculate Wire Size

A wire gauge is a standard unit for measuring the diameter of the wire, and American Wire Gauge (AWG) is the standard used in North America for conductors in electrical systems. Depending on the application, it is essential to use conductors of the proper size for safe and reliable power delivery and to reduce the amount of voltage drop in the system.

An oversized conductor could be a waste of money, and an undersized conductor might not be able to handle the required current flows, which is why choosing the correct wire size is so important.

Minimum Wire Size Formula for Single-Phase Circuits

Use the following formula to calculate the minimum wire size for a DC or single-phase AC circuit:^[1]

A_(m²) = 2 × ρ_(Ω·m) × L_(m) × I_(A) / V_(V)

Where:
A = cross-sectional area in square meters
ρ = conductor resistivity in ohm-meters (Ω·m)
L = length in meters
I = current in amps
V = allowable voltage drop in volts

Thus, the minimum cross-sectional area A for a wire in square meters is equal to the product of two, the conductor resistivity ρ, its length L in meters, and the current I in amps, divided by the allowable voltage drop V in volts.

The resistivity of a copper conductor is 1.7241×10^-8 Ω·m and the resistivity of an aluminum conductor is 2.6548×10^-8 Ω·m.

To convert the resulting cross-sectional area to square millimeters, multiply the result by 1,000,000.

A_(mm²) = _(m²) × 1,000,000

For example, let’s calculate the wire size of a 100-meter-long copper wire for a single-phase circuit when it must supply 30 amps of current with a 10 V voltage drop allowed:

A_(mm²) = 2 × 1.7241×10^-8 Ω·m × 100 m × 30 A / 10 V × 1,000,000
A_(mm²) = 10.3446×10^-6 m² × 1,000,000
A_(mm²) = 10.3446 mm²

By comparing the size to the list of AWG wire sizes in the chart below, we can see that a 7 AWG wire size, which has a cross-sectional area of 10.549 mm², will meet the minimum requirements for this circuit.

Note that these formulas do not account for temperature variation or any national or local electric codes, such as the National Electric Code, that might require a larger gauge conductor, depending on the application.^[2] You can use our wire ampacity calculator to confirm the conductor supports the current requirements for the application.

Always consult a qualified electrical professional before installing or altering electrical wiring or components.

Minimum Wire Size Formula for Three-Phase Circuits

The formula to calculate the wire size for a three-phase AC circuit is slightly different:

A_(m²) = √3 × ρ_(Ω·m) × L_(m) × I_(A) / V_(V)

The minimum cross-sectional area A for a wire in square meters is equal to the product of the square root of three, the conductor resistivity ρ, its length L in meters, and the current I in amps, divided by the allowable voltage drop V in volts.

Solving the same example as before, but for a three-phase circuit, we get:

A_(mm²) = √3 × 1.7241×10^-8 Ω·m × 100 m × 30 A / 10 V × 1,000,000
A_(mm²) = 8.9586×10^-6 m² × 1,000,000
A_(mm²) = 8.9586 mm²

By comparing the size to the list of AWG wires, we can see that a 7 AWG wire size will still meet the minimum requirements for this circuit.

To find the gauge of wire required, use the chart below to find the wire gauge with the necessary cross-sectional area.

How to Calculate a Wire’s Area and Diameter

You can calculate the wire size, diameter, and cross-sectional area using the wire gauge and a few simple formulas.

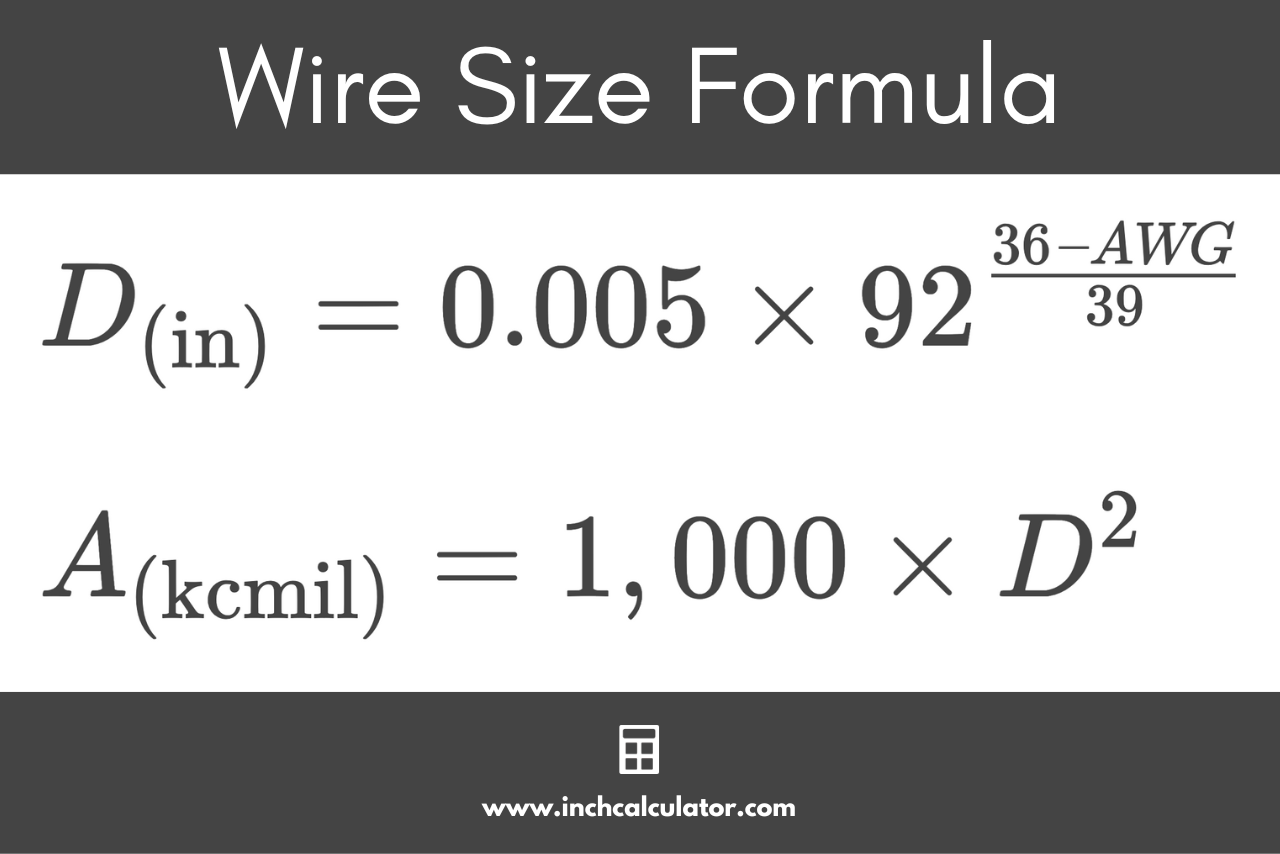
Wire size formulas to calculate the diameter and cross-sectional area of a wire.

Wire Diameter Formula

The formula to find the diameter of the wire in inches is:

D_(in) = 0.005 × 92^{(36 – AWG) ÷ 39}

The formula to find the diameter of the wire in millimeters is:

D_(mm) = 0.127 × 92^{(36 – AWG) ÷ 39}

Steps to Find the Diameter of a Conductor

First – find the exponent in the equation by subtracting the wire gauge from 36 and then dividing by 39.

Finding the exponent for 00, 000, and 0000 gauge wire is slightly different. Substitute -1, -2, and -3 for the gauge in the formula above instead of the AWG value.

Second – find 92 to the power calculated in the previous step.

Third – multiply the value from the second step by either .005 inches or .127 mm to find the diameter of the wire in inches or millimeters, respectively.

Wire Cross-Sectional Area Formula

The formula to find the cross-sectional area of the wire in kilo-circular mils, or kcmil, is:

A_(kcmil) = 1,000 × D_(in)²

The formula to find the cross-sectional area of the wire in square millimeters is:

A_(mm²) = π / 4 × D_(mm)²

Steps to Find the Cross-Sectional Area of a Conductor

First – find the diameter of the wire. Use the formula above to calculate the width if the AWG is known.

Second – multiply the diameter by 1,000 to find the area in kcmil or by (3.1415 ÷ 4) to calculate square millimeters.

Wire Gauge Chart

Chart showing the wire size, diameter, cross-sectional area, and resistances for conductors of each gauge. Resistance values assume a copper conductor at 20 °C (68 °F) with a resistivity of 1.7241×10^-8 Ω·m.
AWG	Diameter		Cross-Sectional Area		Resistance
AWG	(inches)	(mm)	(kcmil)	(mm²)	Ohms per 1,000 ft	Ohms per 1,000 m
0000 (4/0)	0.46	11.684	211.6	107.22	0.049	0.1608
000 (3/0)	0.4096	10.405	167.81	85.029	0.0618	0.2028
00 (2/0)	0.3648	9.266	133.08	67.431	0.0779	0.2557
0 (1/0)	0.3249	8.251	105.53	53.475	0.0983	0.3224
1	0.2893	7.348	83.693	42.408	0.1239	0.4066
2	0.2576	6.544	66.371	33.631	0.1563	0.5127
3	0.2294	5.827	52.635	26.67	0.197	0.6464
4	0.2043	5.189	41.741	21.151	0.2485	0.8152
5	0.1819	4.621	33.102	16.773	0.3133	1.028
6	0.162	4.115	26.251	13.302	0.3951	1.296
7	0.1443	3.665	20.818	10.549	0.4982	1.634
8	0.1285	3.264	16.51	8.366	0.6282	2.061
9	0.1144	2.906	13.093	6.634	0.7921	2.599
10	0.1019	2.588	10.383	5.261	0.9988	3.277
11	0.0907	2.305	8.234	4.172	1.26	4.132
12	0.0808	2.053	6.53	3.309	1.588	5.211
13	0.072	1.828	5.178	2.624	2.003	6.571
14	0.0641	1.628	4.107	2.081	2.525	8.285
15	0.0571	1.45	3.257	1.65	3.184	10.448
16	0.0508	1.291	2.583	1.309	4.015	13.174
17	0.0453	1.15	2.048	1.038	5.063	16.612
18	0.0403	1.024	1.624	0.823	6.385	20.948
19	0.0359	0.9116	1.288	0.6527	8.051	26.415
20	0.032	0.8118	1.022	0.5176	10.152	33.308
21	0.0285	0.7229	0.8101	0.4105	12.802	42.001
22	0.0253	0.6438	0.6424	0.3255	16.143	52.962
23	0.0226	0.5733	0.5095	0.2582	20.356	66.784
24	0.0201	0.5106	0.404	0.2047	25.668	84.213
25	0.0179	0.4547	0.3204	0.1624	32.367	106.19
26	0.0159	0.4049	0.2541	0.1288	40.814	133.9
27	0.0142	0.3606	0.2015	0.1021	51.466	168.85
28	0.0126	0.3211	0.1598	0.081	64.897	212.92
29	0.0113	0.2859	0.1267	0.0642	81.833	268.48
30	0.01	0.2546	0.1005	0.0509	103.19	338.55
31	0.008928	0.2268	0.0797	0.0404	130.12	426.9
32	0.00795	0.2019	0.0632	0.032	164.08	538.32
33	0.00708	0.1798	0.0501	0.0254	206.9	678.8
34	0.006305	0.1601	0.0398	0.0201	260.9	855.96
35	0.005615	0.1426	0.0315	0.016	328.98	1,079.3
36	0.005	0.127	0.025	0.0127	414.84	1,361
37	0.004453	0.1131	0.0198	0.01	523.1	1,716.2
38	0.003965	0.1007	0.0157	0.007967	659.62	2,164.1
39	0.003531	0.0897	0.0125	0.006318	831.77	2,728.9
40	0.003145	0.0799	0.009888	0.00501	1,048.8	3,441.1

Also, see our electricity cost calculator or our lighting cost calculator before you plan your next electrical project.

Wire Size Calculator

Have a Question or Feedback?